Chinese remainder theorem
The Chinese remainder theorem is a result about congruences in number theory and its generalizations in abstract algebra.
In its most basic form it concerned with determining n, given the remainders generated by division of n by several numbers. For example what is the single lowest number if repeatedly divided by 3 gives a remainder of 2; when divided by 5 gives a remainder of 3; and when divided by 7 gives a remainder of 2?
Contents |
Theorem statement
The original form of the theorem, contained in a third-century AD book Sun Zi suanjing (孫子算經 The Mathematical Classic by Sun Zi) by Chinese mathematician Sun Tzu and later republished in a 1247 book by Qin Jiushao, the Shushu Jiuzhang (數書九章 Mathematical Treatise in Nine Sections) is a statement about simultaneous congruences (see modular arithmetic).
Suppose n1, n2, …, nk are positive integers which are pairwise coprime. Then, for any given sequence of integers a1,a2, …, ak, there exists an integer x solving the following system of simultaneous congruences.
Furthermore, all solutions x of this system are congruent modulo to the product N = n1n2…nk.
Hence  for all
for all 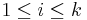 , if and only if
, if and only if 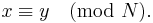
Sometimes, the simultaneous congruences can be solved even if the ni's are not pairwise coprime. A solution x exists if and only if:
All solutions x are then congruent modulo the least common multiple of the ni.
Sun Zi's work contains neither a proof nor a full algorithm. What amounts to an algorithm for solving this problem was described by Aryabhata (6th century; see Kak 1986). Special cases of the Chinese remainder theorem were also known to Brahmagupta (7th century), and appear in Fibonacci's Liber Abaci (1202).
A modern restatement of the theorem in the algebraic language is that for a positive integer  with prime factorization
with prime factorization 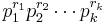 we have the isomorphism between a ring and the direct product of its prime power parts:
we have the isomorphism between a ring and the direct product of its prime power parts:
Existence
Existence can be seen by an explicit construction of  . We will use the notation
. We will use the notation ![[a^{-1}]_b](/2012-wikipedia_en_all_nopic_01_2012/I/6293234fcfb807a6b46ec7f092f01b53.png) to denote the inverse of
to denote the inverse of 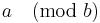 , it is defined exactly when
, it is defined exactly when  and
and 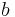 are coprime - the following construction explains why the coprimality condition is needed.
are coprime - the following construction explains why the coprimality condition is needed.
Case of two equations
Given the system (corresponding to  )
)
Since 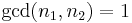 , we have from Bézout's identity
, we have from Bézout's identity
Multiplying both sides by  , we get
, we get
If we take the congruence modulo 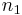 for the right-hand-side expression, it is readily seen that
for the right-hand-side expression, it is readily seen that
But we know that
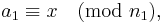
thus this suggests that the coefficient of the first term on the right-hand-side expression should be 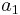 . Similarly, we can show that the coefficient of the second term should be
. Similarly, we can show that the coefficient of the second term should be 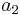 .
.
We can now define the value
and it is seen to satisfy both congruences by reducing. For example
General case
The same type of construction works in the general case of  congruence equations. Let
congruence equations. Let  be the product of every modulus then define
be the product of every modulus then define
and this is seen to satisfy the system of congruences by a similar calculation as before.
A constructive algorithm to find the solution
The following algorithm only applies if the  's are pairwise coprime. (For simultaneous congruences when the moduli are not pairwise coprime, the method of successive substitution can often yield solutions.)
's are pairwise coprime. (For simultaneous congruences when the moduli are not pairwise coprime, the method of successive substitution can often yield solutions.)
Suppose, as above, that a solution is required for the system of congruences:
Again, to begin, the product 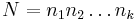 is defined. Then a solution x can be found as follows.
is defined. Then a solution x can be found as follows.
For each i the integers  and
and 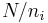 are coprime. Using the extended Euclidean algorithm we can find integers
are coprime. Using the extended Euclidean algorithm we can find integers 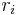 and
and 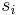 such that
such that 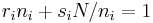 . Then, choosing the label
. Then, choosing the label 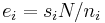 , the above expression becomes:
, the above expression becomes:
Consider 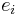 . The above equation guarantees that its remainder, when divided by
. The above equation guarantees that its remainder, when divided by  , must be 1. On the other hand, since it is formed as
, must be 1. On the other hand, since it is formed as  , the presence of N guarantees that it's evenly divisible by any
, the presence of N guarantees that it's evenly divisible by any 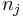 so long as
so long as 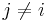 .
.
Because of this, combined with the multiplication rules allowed in congruences, one solution to the system of simultaneous congruences is:
For example, consider the problem of finding an integer x such that
Using the extended Euclidean algorithm for x modulo 3 and 20 [4×5], we find (−13) × 3 + 2 × 20 = 1, i.e. e1 = 40. For x modulo 4 and 15 [3×5], we get (−11) × 4 + 3 × 15 = 1, i.e. e2 = 45. Finally, for x modulo 5 and 12 [3×4], we get 5 × 5 + (−2) × 12 = 1, i.e. e3 = −24. A solution x is therefore 2 × 40 + 3 × 45 + 1 × (−24) = 191. All other solutions are congruent to 191 modulo 60, [3 × 4 × 5 = 60] which means that they are all congruent to 11 modulo 60.
NOTE: There are multiple implementations of the extended Euclidean algorithm which will yield different sets of 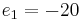 ,
,  , and
, and  . These sets however will produce the same solution i.e. (-20)2+(-15)3+(-24)1=-109=11 modulo 60.
. These sets however will produce the same solution i.e. (-20)2+(-15)3+(-24)1=-109=11 modulo 60.
Statement for principal ideal domains
For a principal ideal domain R the Chinese remainder theorem takes the following form: If u1, ..., uk are elements of R which are pairwise coprime, and u denotes the product u1...uk, then the quotient ring R/uR and the product ring R/u1R× ... × R/ukR are isomorphic via the isomorphism
such that
This map is well-defined and an isomorphism of rings; the inverse isomorphism can be constructed as follows. For each i, the elements ui and u/ui are coprime, and therefore there exist elements r and s in R with
Set ei = s u/ui. Then the inverse of f is the map
such that
Note that this statement is a straightforward generalization of the above theorem about integer congruences: the ring Z of integers is a principal ideal domain, the surjectivity of the map f shows that every system of congruences of the form
can be solved for x, and the injectivity of the map f shows that all the solutions x are congruent modulo u.
Statement for general rings
The general form of the Chinese remainder theorem, which implies all the statements given above, can be formulated for commutative rings and ideals. If R is a commutative ring and I1, ..., Ik are ideals of R which are pairwise coprime (meaning that  for all
for all 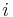 ), then the product I of these ideals is equal to their intersection, and the quotient ring R/I is isomorphic to the product ring R/I1 x R/I2 x ... x R/Ik via the isomorphism
), then the product I of these ideals is equal to their intersection, and the quotient ring R/I is isomorphic to the product ring R/I1 x R/I2 x ... x R/Ik via the isomorphism
such that
Here is a version of the theorem where R is not required to be commutative:
Let R be any ring with 1 (not necessarily commutative) and  be pairwise coprime 2-sided ideals. Then the canonical R-module homomorphism
be pairwise coprime 2-sided ideals. Then the canonical R-module homomorphism 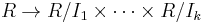 is onto, with kernel
is onto, with kernel  . Hence,
. Hence, 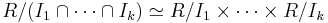 (as R-modules).
(as R-modules).
Applications
- In the RSA algorithm calculations are made modulo n, where n is a product of two large prime numbers p and q. 1024-, 2048- or 4096-bit integers n are commonly used, making calculations in
 very time-consuming. By the Chinese remainder theorem, however, these calculations can be done in the isomorphic ring
very time-consuming. By the Chinese remainder theorem, however, these calculations can be done in the isomorphic ring  instead. Since p and q are normally of about the same size, that is about
instead. Since p and q are normally of about the same size, that is about  , calculations in the latter representation are much faster. Note that RSA algorithm implementations using this isomorphism are more susceptible to fault injection attacks.
, calculations in the latter representation are much faster. Note that RSA algorithm implementations using this isomorphism are more susceptible to fault injection attacks.
- The Chinese remainder theorem may also be used to construct an elegant Gödel numbering for sequences, which is needed to prove Gödel's incompleteness theorems.
- The following example shows a connection with the classic polynomial interpolation theory. Let r complex points ("interpolation nodes")
 be given, together with the complex data
be given, together with the complex data 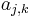 , for all
, for all 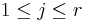 and
and 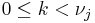 . The general Hermite interpolation problem asks for a polynomial
. The general Hermite interpolation problem asks for a polynomial ![\textstyle P(x)\in \C[x]](/2012-wikipedia_en_all_nopic_01_2012/I/892dd428009cd6c88d21b5684b63e94a.png) taking the prescribed derivatives in each node
taking the prescribed derivatives in each node 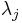 :
:
-
- Introducing the polynomials
 ,
,
- the problem may be equivalently reformulated as a system of
 simultaneous congruences:
simultaneous congruences:
- By the Chinese remainder theorem in the principal ideal domain
![\textstyle\C[x]](/2012-wikipedia_en_all_nopic_01_2012/I/bb457b423b31e79bdfdbde02ca60ed16.png) , there is a unique such polynomial
, there is a unique such polynomial 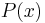 with degree
with degree  . A direct construction, in analogy with the above proof for the integer number case, can be performed as follows. Define the polynomials
. A direct construction, in analogy with the above proof for the integer number case, can be performed as follows. Define the polynomials  and
and 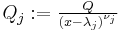 . The partial fraction decomposition of
. The partial fraction decomposition of 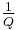 gives r polynomials
gives r polynomials 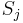 with degrees
with degrees 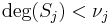 such that
such that
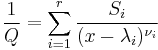 ,
,
- so that
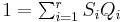 . Then a solution of the simultaneous congruence system is given by the polynomial
. Then a solution of the simultaneous congruence system is given by the polynomial
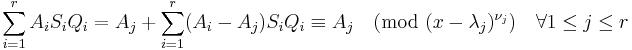 ;
;
- and the minimal degree solution is this one reduced modulo
 , that is the unique with degree less than n.
, that is the unique with degree less than n.
- The Chinese remainder theorem can also be used in Secret sharing, which consists of distributing a set of shares among a group of people who, all together (but no one alone), can recover a certain secret from the given set of shares. Each of the shares is represented in a congruence, and the solution of the system of congruences using the Chinese remainder theorem is the secret to be recovered. Secret Sharing using the Chinese Remainder Theorem uses, along with the Chinese remainder theorem, special sequences of integers that guarantee the impossibility of recovering the secret from a set of shares with less than a certain cardinality.
- The Good-Thomas fast Fourier transform algorithm exploits a re-indexing of the data based on the Chinese remainder theorem. The Prime-factor FFT algorithm contains an implementation.
- Dedekind's theorem on the linear independence of characters states (in one of its most general forms) that if M is a monoid and k is an integral domain, then any finite family
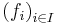 of distinct monoid homomorphisms
of distinct monoid homomorphisms 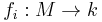 (where the monoid structure on k is given by multiplication) is linearly independent, i. e. every family
(where the monoid structure on k is given by multiplication) is linearly independent, i. e. every family 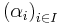 of elements
of elements 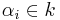 satisfying
satisfying 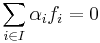 must be equal to the family
must be equal to the family 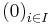 .
.
- Proof using the Chinese Remainder Theorem: First, assume that k is a field (otherwise, replace the integral domain k by its quotient field, and nothing will change). We can linearly extend the monoid homomorphisms
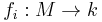 to k-algebra homomorphisms
to k-algebra homomorphisms ![F_i:k\left[M\right]\to k](/2012-wikipedia_en_all_nopic_01_2012/I/e324725799553d97ffdfe19dc9db5bcd.png) , where
, where ![k\left[M\right]](/2012-wikipedia_en_all_nopic_01_2012/I/ac33c8178bdb9267d5a398852f22c40e.png) is the monoid ring of M over k. Then, the condition
is the monoid ring of M over k. Then, the condition 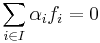 yields
yields 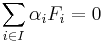 by linearity. Now, we notice that if
by linearity. Now, we notice that if 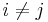 are two elements of the index set I, then the two k-linear maps
are two elements of the index set I, then the two k-linear maps ![F_i:k\left[M\right]\to k](/2012-wikipedia_en_all_nopic_01_2012/I/e324725799553d97ffdfe19dc9db5bcd.png) and
and ![F_j:k\left[M\right]\to k](/2012-wikipedia_en_all_nopic_01_2012/I/62323981f5f90b7c6d15d91ebbdee0f9.png) are not proportional to each other (because if they were, then
are not proportional to each other (because if they were, then 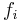 and
and 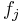 would also be proportional to each other, and thus equal to each other since
would also be proportional to each other, and thus equal to each other since 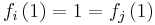 (since
(since 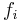 and
and 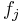 are monoid homomorphisms), contradicting the assumption that they be distinct). Hence, their kernels
are monoid homomorphisms), contradicting the assumption that they be distinct). Hence, their kernels 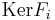 and
and 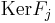 are distinct. Now,
are distinct. Now, 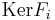 is a maximal ideal of
is a maximal ideal of ![k\left[M\right]](/2012-wikipedia_en_all_nopic_01_2012/I/ac33c8178bdb9267d5a398852f22c40e.png) for every
for every 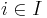 (since
(since ![k\left[M\right] / \mathrm{Ker} F_i\cong F_i\left(k\left[M\right]\right)=k](/2012-wikipedia_en_all_nopic_01_2012/I/7c8e9c5d43c9d3b6eb46183474a52436.png) is a field), and the ideals
is a field), and the ideals 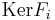 and
and 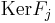 are coprime whenever
are coprime whenever 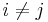 (since they are distinct and maximal). The Chinese Remainder Theorem (for general rings) thus yields that the map
(since they are distinct and maximal). The Chinese Remainder Theorem (for general rings) thus yields that the map
- given by
 for all
for all ![x\in k\left[M\right]](/2012-wikipedia_en_all_nopic_01_2012/I/c8667492930f1eb307e1f464f6d87677.png)
- is an isomorphism, where
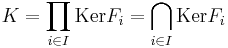 . Consequently, the map
. Consequently, the map
- given by
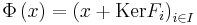 for all
for all ![x\in k\left[M\right]](/2012-wikipedia_en_all_nopic_01_2012/I/c8667492930f1eb307e1f464f6d87677.png)
- is surjective. Under the isomorphisms
![k\left[M\right] / \mathrm{Ker} F_i\to F_i\left(k\left[M\right]\right)=k](/2012-wikipedia_en_all_nopic_01_2012/I/9b6b3eac7720cd6c1d91ee111e319931.png) , this map
, this map 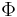 corresponds to the map
corresponds to the map
- given by
 for every
for every ![x\in k\left[M\right]](/2012-wikipedia_en_all_nopic_01_2012/I/c8667492930f1eb307e1f464f6d87677.png) .
.
- Now,
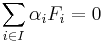 yields
yields 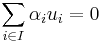 for every vector
for every vector 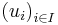 in the image of the map
in the image of the map 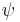 . Since
. Since 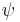 is surjective, this means that
is surjective, this means that 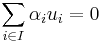 for every vector
for every vector 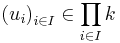 . Consequently,
. Consequently, 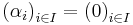 , qed.
, qed.
Non-commutative case: a counter-example
The Chinese remainder theorem does not hold in the non-commutative case. Consider the ring R of non-commutative real polynomials in x and y. Let I be the principal two-sided ideal generated by x and J the principal two-sided ideal generated by 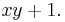 Then
Then 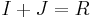 but
but 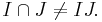
Proof
Observe that I is formed by all polynomials with an x in every term and that every polynomial in J vanishes under the substitution  . Consider the polynomial
. Consider the polynomial 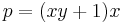 . Clearly
. Clearly 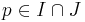 . Define a term in R as an element of the multiplicative monoid of R generated by x and y. Define the degree of a term as the usual degree of the term after the substitution
. Define a term in R as an element of the multiplicative monoid of R generated by x and y. Define the degree of a term as the usual degree of the term after the substitution 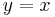 . On the other hand, suppose
. On the other hand, suppose 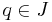 . Observe that a term in q of maximum degree depends on y otherwise q under the substitution
. Observe that a term in q of maximum degree depends on y otherwise q under the substitution  can not vanish. The same happens then for an element
can not vanish. The same happens then for an element  . Observe that the last y, from left to right, in a term of maximum degree in an element of
. Observe that the last y, from left to right, in a term of maximum degree in an element of 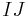 is preceded by more than one x. (We are counting here all the preceding xs. e.g. in
is preceded by more than one x. (We are counting here all the preceding xs. e.g. in 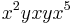 the last y is preceded by
the last y is preceded by 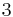 xs.) This proves that
xs.) This proves that 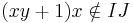 since that last y in a term of maximum degree (
since that last y in a term of maximum degree (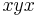 ) is preceded by only one x. Hence
) is preceded by only one x. Hence 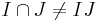 .
.
On the other hand, it is true in general that 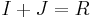 implies
implies 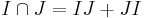 . To see this, note that
. To see this, note that 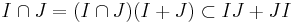 , while the opposite inclusion is obvious. Also, we have in general that, provided
, while the opposite inclusion is obvious. Also, we have in general that, provided  are pairwise coprime two-sided ideals in R, the natural map
are pairwise coprime two-sided ideals in R, the natural map
is an isomorphism. Note that 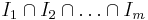 can be replaced by a sum over all orderings of
can be replaced by a sum over all orderings of  of their product (or just a sum over enough orderings, using inductively that
of their product (or just a sum over enough orderings, using inductively that 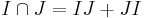 for coprime ideals
for coprime ideals 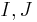 ).
).
See also
- Covering system
- Hasse principle
- Residue number system
- Secret sharing using the Chinese remainder theorem
References
- Donald Knuth. The Art of Computer Programming, Volume 2: Seminumerical Algorithms, Third Edition. Addison-Wesley, 1997. ISBN 0-201-89684-2. Section 4.3.2 (pp.286–291), exercise 4.6.2–3 (page 456).
- Thomas H. Cormen, Charles E. Leiserson, Ronald L. Rivest, and Clifford Stein. Introduction to Algorithms, Second Edition. MIT Press and McGraw-Hill, 2001. ISBN 0-262-03293-7. Section 31.5: The Chinese remainder theorem, pp.873–876.
- Laurence E. Sigler (trans.) (2002). Fibonacci's Liber Abaci. Springer-Verlag. pp. 402–403. ISBN 0-387-95419-8.
- Kak, Subhash (1986), "Computational aspects of the Aryabhata algorithm", Indian Journal of History of Science 21 (1): 62–71, http://www.ece.lsu.edu/kak/AryabhataAlgorithm.pdf.
- Thomas W. Hungerford (1974). Algebra. Springer-Verlag. pp. 131–132. ISBN 0-387-90518-9.
- Cunsheng Ding, Dingyi Pei, and Arto Salomaa (1996). Chinese Remainder Theorem: Applications in Computing, Coding, Cryptography. World Scientific Publishing. pp. 1–213. ISBN 981-02-2827-9.
External links
- "Chinese Remainder Theorem" by Ed Pegg, Jr., Wolfram Demonstrations Project, 2007.
- Weisstein, Eric W., "Chinese Remainder Theorem" from MathWorld.
- C# program and discussion at codeproject
- University of Hawaii System CRT by Lee Lady
- Full text of the Sunzi Suanjing (Chinese) - Chinese Text Project
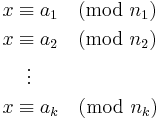
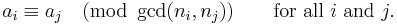
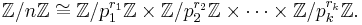
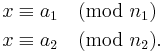
![n_2 [n_2^{-1}]_{n_1} %2B n_1 [n_1^{-1}]_{n_2} = 1.](/2012-wikipedia_en_all_nopic_01_2012/I/bf67ad32d9f755a8ecc929bdfb53de67.png)
![x = x n_2 [n_2^{-1}]_{n_1} %2B x n_1 [n_1^{-1}]_{n_2}.](/2012-wikipedia_en_all_nopic_01_2012/I/493f7c007ffe7923760148aa16b8f1b9.png)
![x n_2 [n_2^{-1}]_{n_1} %2B x n_1 [n_1^{-1}]_{n_2} \equiv x 1 %2B x 0 [n_1^{-1}]_{n_2} \equiv x \pmod {n_1}.](/2012-wikipedia_en_all_nopic_01_2012/I/61b712cfacea62c607bf123a34118ec9.png)
![x�:= a_1 n_2 [n_2^{-1}]_{n_1} %2B a_2 n_1 [n_1^{-1}]_{n_2}](/2012-wikipedia_en_all_nopic_01_2012/I/9989bde98c7f37e6fa2fa42c3622d37d.png)
![a_1 n_2 [n_2^{-1}]_{n_1} %2B a_2 n_1 [n_1^{-1}]_{n_2} \equiv a_1 1 %2B a_2 0 [n_1^{-1}]_{n_2} \equiv a_1 \pmod {n_1}.](/2012-wikipedia_en_all_nopic_01_2012/I/42feffb6f53e52eea7d88cbb6a856a4c.png)
![x�:= \sum_{i} a_i \frac{N}{n_i} \left[\left(\frac{N}{n_i}\right)^{-1}\right]_{n_i}](/2012-wikipedia_en_all_nopic_01_2012/I/7900728a23224d40167ad92714699ac2.png)
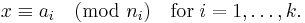
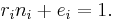
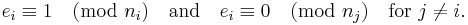
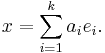
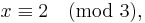
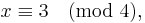
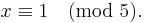
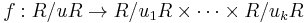
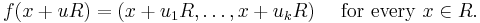
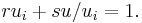
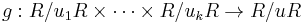
![g(a_1%2Bu_1R,\ldots ,a_k%2Bu_kR)=
\left( \sum_{i=1}^k a_i \frac{u}{u_i} \left[\left(\frac{u}{u_i}\right)^{-1}\right]_{u_i} \right) %2B uR \quad\mbox{ for all }a_1,\ldots,a_k\in R.](/2012-wikipedia_en_all_nopic_01_2012/I/dee8d84f2293e78bf93542f068a0038d.png)
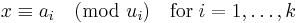
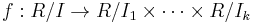

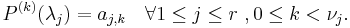
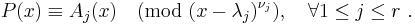
![\phi: k\left[M\right] / K\to \prod_{i\in I}k\left[M\right] / \mathrm{Ker} F_i](/2012-wikipedia_en_all_nopic_01_2012/I/e521b8523019c5136b45d4d436512910.png)
![\Phi: k\left[M\right]\to \prod_{i\in I}k\left[M\right] / \mathrm{Ker} F_i](/2012-wikipedia_en_all_nopic_01_2012/I/83e72ca25e340e3db003765a3d5b3333.png)
![\psi: k\left[M\right]\to \prod_{i\in I}k](/2012-wikipedia_en_all_nopic_01_2012/I/9b3f9ea364213f9fdabcff978ec00265.png)
